Boat races involve sailing around a series of marks. The boat that gets around all the marks fastest wins.

On my computer I’ve built some boats, trained a few tacticians and even created wind – everything we need for a race! I’ve laid an upwind mark for my tacticians to aim at but I’ve not taught any of them how to go around it, so let’s say the winner is the boat that makes it furthest towards the wind before time runs out.

The video below helps illustrate what we are looking at. It shows some boats starting a race then cuts to them tacking into the wind.

Wind

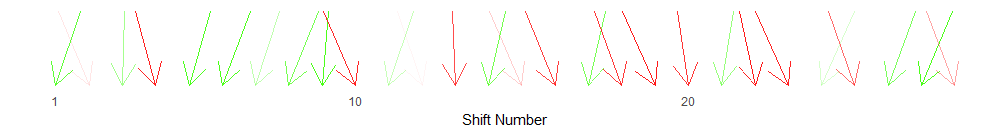
My model wind blows at a constant strength but changes direction to simulate the wind shifts that govern tactics during a real race. I picked the direction from a normal distribution and fiddled with the parameters to get something that looks nice. I’ve plotted the wind shifts below, the first shift to reach the boats is on the left and the last is on the right. Darker shifts last longer than pale ones.

You can shorten the distance sailed upwind by playing the shifts. Generally, if the wind swings left you want to go right and vice-versa. You catch the shifts by tacking but this comes at a cost. Tacking is an aerodynamic disaster, everything flaps, there’s lots of drag, the boat slows down and it takes time to get back up to speed. Having a good crew helps but there is no escaping the aerodynamic shit show that happens during a tack. This video shows a well drilled crew performing a good tack, quickly followed by another after something breaks.

Tactician

The canny tactician thinks carefully about each tack, balancing the loss in speed with the potential gain it awards.

I’ve given each tactician an identical boat whose speed hits 0 when it tacks and accelerates until it reaches top speed. Some boats have good crews who can accelerate faster than the bad crews, but all the boats have the same top speed and sail at the same angle to the wind.

Everyone starts on Starboard and sails into wind coming from the North (top of the screen) following one of six rules:

Sail left.
Sail right.
Bang the corner:
1. Sail in one direction until you think you can lay the mark. Tack on the lay-line.
Tack every change (twitchy).
1. This tactician doesn’t know the mean wind direction so they tack on every change. If the wind goes left from where it is now, the tactician goes onto port. If in doubt they sail on starboard.
Tack every shift.
1. If the wind goes to the left of mean, tack on to port and vice-versa.
Tack the big shifts.
1. Tack shifts bigger than 5 degrees.

Results

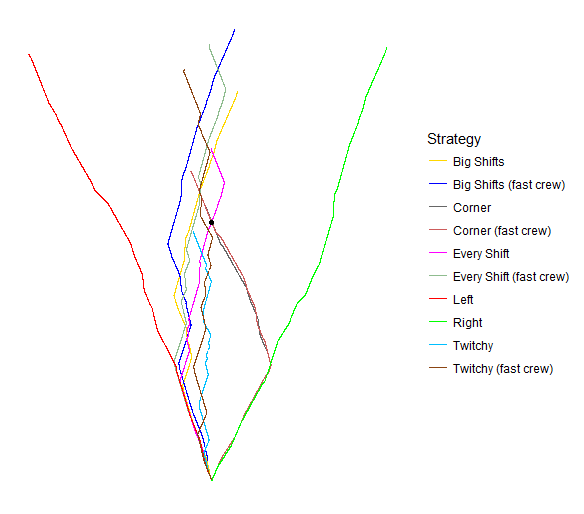
The outcomes are in the plot below. The boats banging the corners aim for the black mark, upwind and North of the start.

The boat that only tacks on big shifts with a fast crew wins, followed by the boat that tacks on every shift. In 3rd and 4th places are boats whose strategies don’t rely on tacking and the twitchy boat follows up the rear. The pattern is repeated amongst the slower crews.

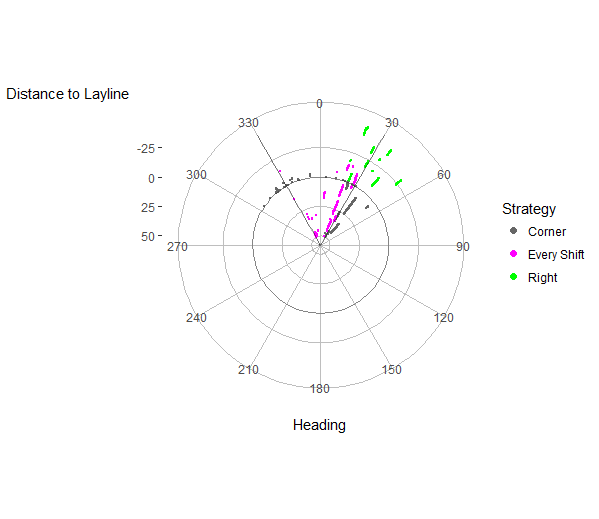
The polar plot below explores how the strategies which bang the corner, tack on every shift and doggedly sail right behave in more detail. The lay-line is highlighted with the slightly darker ring and the boat’s heading is with reference to North at the top of the screen.

Up to the lay-line, the headings of the boats sailing right and into the corner are directly governed by the wind shifts – if the wind shifts left, the boats point further left and vice-versa. The boat tacking every shift goes in the direction of the best shift. The boat tacking every shift doesn’t reach the lay-line until it’s nearly on top of the mark, whereas the boat banging the corner reaches the lay-line quite early on and once on it, gets a series of left hand shifts lifting the boat over the lay-line.

This model shouldn’t be taken as anything too meaningful – every element in it was arbitrarily chosen by me. In this form it’s just a bit of fun but I could add meaning to this model by:

Tying it to the real world i.e use some real wind and boat data
Varying the parameters to create dimensionless comparison statements - how does a boat with high top speed and a slow crew compare to a slower boat with a faster crew in shifty conditions? How stable does the wind need to be before the advantage changes? How much faster does the fast boat need to be? How much faster do the fast crew need to be?

Having said that, my model helps highlight a few things which I believe to be true. More information is better. The Twitchy tactician does worse than the those with knowledge of the mean wind direction.

There is an endless avenue of further exploration that I could incorporate into my model but for now, thank you.